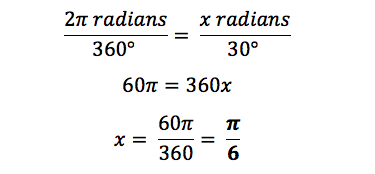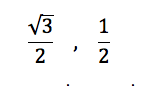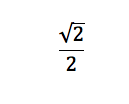Irvine Math Tutoring: The Unit Circle


The Unit Circle is a staple of trigonometry and precalculus classes. It is a circle with a radius of one that is centered at the origin of a two-dimensional coordinate system. Essentially the simplest circle that we can put on our grid – book your private Irvine math tutor today.
Nearly every class will require students to memorize specific angles and they’re coordinates on this circle. For example, the “top” of the circle is at 90° (the angle is measured from the right side of the x-axis, or the “East” stem if you think of it as a compass) which is the point (0 , 1) since it is straight up and the unit circle has a radius of one. Similarly, we get (0, -1) at 270° at the bottom of the circle. The harder memorization comes in when you look at some of the points that are don’t lie perfectly on our axes. See an image of a typical unit circle below.

image taken from Wikipedia, submitted by Jim.belk
Here, we see the points we mentioned, but also a lot of pi symbols, radicals, and many fractions. This image can look quite daunting since most teachers expect you to be able to draw it yourself on command. So, let’s dissect how to learn it more easily with much less memorization.
First, we need to know how to use radians (a way to measure angles without degrees). We won’t get into why radians are the way they are in this post, but you understand them on the unit circle. You’ll need to know two facts:
A circle is 360°
A circle is 2π radians
With these two facts, we can convert between the two with some dimensional analysis. It’s like how knowing that 12 inches is 1 foot allows you to figure out that 4 feet is 48 inches. For some examples, here is how to find 30° in radians:
Here we set up the fractions since we know that 2π is the same as 360°. You cross multiply and divide to find x, simplifying the fraction at the end. Here is the same concept except converting from radians to degrees. Let’s say we have π/4 and want to find it in degrees:
Here we had some more fractions to work with, but the pis cancel out to give us 45°.
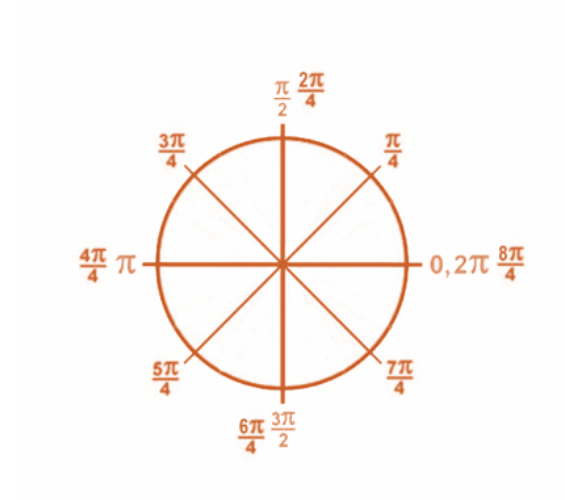
Now back to the unit circle. The unit circle is better memorized as two circles instead of one. On one circle they count by 30° increments (which we just learned is equal to π/6 radians) and on the other, we count by 45° increments (which we also just learned is equal to π/4 radians). Here is circle number one:
Notice the bold terms. They all have a denominator of 6. This circle corresponds to the blue lines we see on Wikipedia circle. But notice how much easier it is to memorize in increments of π/6. One π/6, Two π/6, Three π/6, Four π/6, etc. up until all the way around the circle is Twelve π/6. The unit circle is just simplifying the fractions! 12 π/6 is just 2π since 12/6 = 2. Just count the π/6’s around the circle and simplify the fractions. Much simpler than memorizing all of those fractions.
Now that we know the angles of the unit circle, we have to learn the coordinates at each angle. The ones on the corners aren’t bad since those are just variations of -1, 0, and 1 and we can tell what the coordinate pair should be. For the remaining 8 points, here are the only two numbers we need to memorize:
Again, we won’t go into why these are the numbers since we’re just focused on memorization. Notice here that they both have a denominator of 2. Then, notice that √3 is larger than 1. Every coordinate point will be a combination of these points, so just look for which side is bigger. If the x side looks bigger (like in π/6), then the x side gets the √3/2 and the y side gets the 1/2. For 10π/6, notice that the longer side is in the y-direction and is going down. This means the y coordinate get the √3/2 and it is negative: (1/2, -√3/2).
Notice now that the bold terms are all with a denominator of 4. Here we count by π/4’s instead of π/6’s. This circle corresponds to the red lines on the regular unit circle. Here we count increments of π/4 until we get to 8π/4 which is our full circle of two pi. Memorize that these are the two circles that are put on top of each other for the full unit circle. Both are just counting until you get to 2π.
Now we’ll learn the coordinate points for this circle. The “corners are still the same as the blue circle ((1,0), (0,1), (-1,0), and (0,-1)), and we only have one number to memorize for the diagonal angles in between:
All of the coordinates for these angles on the unit circle will be √2/2 for both x and y. Just don’t forget to include the negative signs when necessary. So, for example, 3π/4 will be (-√2/2, √2/2) and 5π/4 will be (-√2/2, -√2/2).
If you can keep these two circles separate in your head it will significantly help you when drawing your own: and without the brute force memorization of every reduced fraction that many teachers suggest. Notice too that the diagonals of the orange circle fit perfectly between the diagonals of the blue circle since 45° is halfway between 30° and 60°.
Though memorization is still necessary, hopefully this guide will save you from mindlessly cramming and consequently forgetting your unit circle as you delve deeper into trigonometry.
From trigonometry to statistics, our private Irvine math tutors are here to help. Call TutorNerds today to book you Irvine math tutor.
Michael C. is currently a private math, science, and standardized test tutor with TutorNerds in Irvine and Anaheim.
replace logoAll blog entries, with the exception of guest bloggers, are
100% Satisfaction Guarantee
You’ll love your tutor, or you don’t pay.

We will evaluate your situation and answer any questions. We will then individually match you with one of our tutors. Your tutor will recommend the best strategies for you based on your goals. You’ll work with the same tutor ongoing and you can schedule directly with your tutor.
100% Satisfaction Guarantee – You’ll love your tutor, or you don’t pay
| Cookie | Duration | Description |
|---|---|---|
| cookielawinfo-checkbox-analytics | 11 months | This cookie is set by GDPR Cookie Consent plugin. The cookie is used to store the user consent for the cookies in the category "Analytics". |
| cookielawinfo-checkbox-functional | 11 months | The cookie is set by GDPR cookie consent to record the user consent for the cookies in the category "Functional". |
| cookielawinfo-checkbox-necessary | 11 months | This cookie is set by GDPR Cookie Consent plugin. The cookies is used to store the user consent for the cookies in the category "Necessary". |
| cookielawinfo-checkbox-others | 11 months | This cookie is set by GDPR Cookie Consent plugin. The cookie is used to store the user consent for the cookies in the category "Other. |
| cookielawinfo-checkbox-performance | 11 months | This cookie is set by GDPR Cookie Consent plugin. The cookie is used to store the user consent for the cookies in the category "Performance". |
| viewed_cookie_policy | 11 months | The cookie is set by the GDPR Cookie Consent plugin and is used to store whether or not user has consented to the use of cookies. It does not store any personal data. |